Have you ever taught a lesson and realized too few of your students learned what you taught? You’re not alone! We’ve experienced this numerous times in our years as classroom teachers and in our current roles. In this blog post, Gabe shares his experiences from teaching and his role as elementary school principal. Together, we share insights from our collaboration and shared experiences.
Gabe:
After 20 years of working with elementary school children, I finally started to find answers to the pedagogical questions nagging me since my first days teaching mathematics. I also realized how powerful it is to expand my understanding of math concepts beyond the narrow scope I’d experienced — and taught — my entire life.
As a systems thinker, I’d constrained math instruction to a series of prescribed steps, completely disconnected from the mathematical concept. I streamlined tasks into a sequence that could be introduced and modeled — steps that students could rehearse as many times as necessary. Most lessons were a version of,
“Here’s the lesson objective, relevant vocabulary, and the steps we need to follow. Now, we will practice these steps as many times as we can before lunch.”
Over the past two years, I began to emerge from my constrained view of math instruction. More than any other aspect of teaching, math instruction is the domain I would revise if I could revisit my years as a classroom teacher. Now, as the principal of an elementary school, my role is to be the lead learner. To me, this means I must first experience the steps it takes to learn new instructional strategies and implement them in classrooms at various grade levels. To do this, I schedule the time to co-teach math lessons in classrooms at the school where I work.
Developing a Student-Centered Approach to Math
Regardless of the role one has at a school site, the opportunities to continue learning and growing abound. While we’ve both spent 20 years teaching, the reality is, we’ve spent 20 years learning, too. We’re still developing our ability to learn from teaching.
It starts by being proactive about learning, and thinking about ways you can learn from practice, both your own practice and the practice of others. The formal professional development opportunities scheduled for teachers may not always address the questions on your mind, so be cognizant of the questions and interests that develop as you pursue your own learning. These are likely the same areas where your students will benefit from your guidance.
Developing a student-centered approach to math has required numerous steps.
Step One: Build Background Knowledge of Strategies Beyond the Traditional Algorithm
- Reading books and papers such as Van De Walle’s 10th edition and articles from NCTM’s Teaching Children Mathematics.
- Another useful tool is classroom video. Consider watching with a lens for understanding the mathematical ideas inherent in the video. One that resonated with us recently was Teaching Channel‘s Reasoning About Division. In this video, a teacher and her students explore division. She presents a number string, encouraging students to use what they know about place value and reasoning to make sense of the operation with various quantities. The student thinking that’s evident here is quite different than a traditional listing of steps.
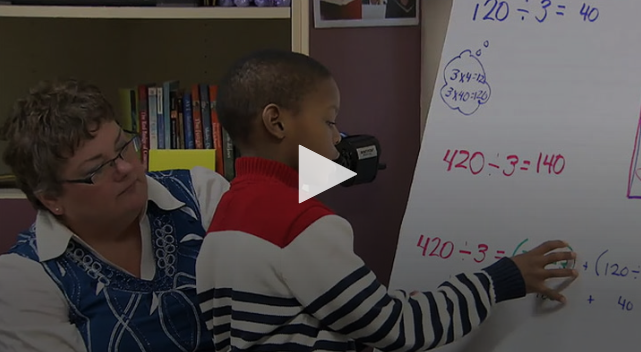
VIDEO: Reasoning About Division
Step Two: Planning for Instruction
- Beyond planning which problems students will solve, solve the problems in multiple ways ahead of time. Paying attention to your own questions and realizations throughout each strategy is helpful in anticipating strategies students may use. For example, when preparing to work with kindergarten students, consider ways to decompose 10 into pairs (K.OA.3). Sketch out various combinations on scratch paper and ask yourself: How might students approach this problem? Will they randomly think of pairs or approach it systematically, for example, beginning with 1 and working their way to 10?
- Doing the math and anticipating in advance is helpful in planning teacher moves to elicit particular ideas. For example, when asking students to share combinations of 10, consider how student ideas are recorded, and whether this will support them in seeing the structure.
Step Three: Make Math Problems Memorable
How often does our traditional math instruction produce problems that resonate with students? No one remembers solving 12 x 3 if it’s one of the twenty-eight practice problems on a page, but contextualized in a real-world example and explored through numerous strategies, 12 x 3 could be the expression from a memorable challenge. This idea resonated with us from the Reasoning About Division video. In it, the teacher provides context and gives the students a True/False equation:
80 skittles divided by four kids is the same amount as 80 skittles divided by two kids and 80 skittles divided by two kids.
- Students share various ways of thinking about the problem. One in particular resonated with us, when a student said, “I think it’s false because if you do 80 divided by 2, you’ve already split up all the skittles; all the skittles are already gone.” The context contributed to student sense-making and likely made the problem more memorable.
- The work within this video was recorded on chart paper, potentially creating an artifact of the experience that could then be revisited. This provided an image of what a rich, memorable student learning experience might look like and sound like. How can we ensure students under our watch have similar experiences?
Step Four: Let Go of Control and Accept Uncertainty
To embrace the possibilities of student-centered math, let go of trying to follow a precise script. The paradox is you plan more, but end up with less certainty about what direction the lesson will take.
- Start planning by identifying what concept students will need to learn, and plan to include thought-provoking problems.
- Plan how you hope the dialogue will go, but adopt a philosophy of being prepared to be surprised. Open yourself up as a learner, learning with and from the students you work with. Learn from the work of teaching.
Step Five: See Math Through Students’ Eyes
Effectively teaching various strategies requires the teacher to show empathy for the students.
- Develop a healthy respect for the cognitive challenge of the concept at your students’ developmental levels. Students learning the content of their own grade level should be given the same deference as any college-level mathematicians.
Reflecting On Process and Learning: Collaboration is Instrumental
Want to try out new things and think about how you can learn in and from practice?
- Begin with the buddy system.
- Select a lesson that piques your interest — number strings, a new model, or something that students have responded to in unexpected ways.
- Pre-read all necessary materials and resources.
- Identify if there’s a particular number or sequence to include to seed a specific idea.
- Plan with a partner prior to the lesson.
- Predict where students will struggle, succeed, and how you’ll respond to each.
- Co-teach the lesson, and take notes along the way.
- Allow ample time to debrief after teaching.
- Pay attention to the ways you were prepared for student thinking and learn from the ways you were not.
As you debrief, ask yourselves the following questions:
- What surprised you during the lesson?
- Was there a student response you hadn’t expected?
- Was there evidence of the students making progress toward the learning goal?
How do you reflect on process, learning, and growth? What role does collaboration play in how you learn from practice?






